Lesson .

Cloud Computing: A 'Pi in the Sky' Math Challenge

Last Updated: June 20, 2025

Subject

Math

Grade Levels

6-12

Time Required

Under 30 mins

Overview

The "Pi in the Sky" math challenge gives students a chance to take part in recent discoveries and upcoming celestial events, all while using math and pi just like NASA scientists and engineers. In this problem from the set, students use the mathematical constant pi to calculate the amount of potential rainfall in a cloud.

Materials

Pi in the Sky 6: Cloud Computing worksheet – Download PDF
Pi in the Sky 6 answer key – Download PDF

Background

This 3D image from the MISR instrument on NASA's Terra satellite shows Hurricane Florence as it approached the eastern coast of the United States on Sept. 13, 2018. › Full image and caption.

Credit: NASA/GSFC/LaRC/JPL-Caltech, MISR Team

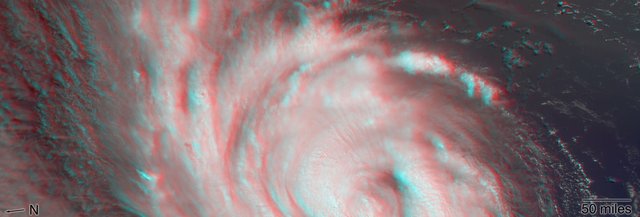
The Terra satellite uses the nine cameras on its Multi-Angle Imaging SpectroRadiometer, or MISR, instrument to provide scientists with unique views of Earth, returning data about atmospheric particles, land-surface features and clouds. Estimating the amount of water in a cloud and the potential for rainfall is serious business. Knowing how much rain may fall in a given area can help residents and first responders prepare for emergencies like flooding and mudslides. In Cloud Computing, students can use their knowledge of pi and geometric shapes to estimate the amount of water contained in a cloud.

Procedures

Cloud Computing

The MISR instrument on NASA’s Terra satellite has nine cameras that view Earth from different angles to study features on the surface and in the atmosphere in 3D. One of MISR’s tasks is to collect measurements of clouds, which are full of liquid water or ice. Scientists can use the measurements to estimate how much water is in a cloud. Imagine MISR flies over a cloud that from directly overhead looks like a circle, 10 km across. From the side, it looks like a soup can, indicating it’s roughly the shape of a right cylinder.

Given that the cloud’s top and height measure 16 km combined, calculate the approximate volume of the cloud in cubic kilometers.

Given the liquid water content of a typical puffy cumulus cloud, calculate the total amount of water in the cloud.

If all the water in the cloud fell as rain, how many Olympic size swimming pools could it fill?

Cumulus cloud liquid water content = 500,000 kg/km³ Olympic swimming pool volume = 2,500 m³ Water density = 1,000 kg/m³

Illustration of clouds floating over the land with a large cloud in the middle showing an outline of a cylinder and a measurement of 10 km. The terra satellite is flying above with arrays from its nine cameras pointing down at the land.

› Download PDF.

Credit: NASA/JPL-Caltech/Kim Orr

Assessment

Infographic answer key for all of the Pi in the Sky 6 graphics and problems

Extensions

Participate

educators.

Pi Day Challenge Lessons

Here's everything you need to bring the NASA Pi Day Challenge into the classroom.

students.

Slideshow: NASA Pi Day Challenge

The entire NASA Pi Day Challenge collection can be found in one, handy slideshow for students.

Join the conversation and share your Pi Day Challenge answers with @NASAJPL_Edu on social media using the hashtag #NASAPiDayChallenge

Blogs and Features

students.

How Many Decimals of Pi Do We Really Need?

While you may have memorized more than 70,000 digits of pi, world record holders, a JPL engineer explains why you really only need a tiny fraction of that for most calculations.

students.

Slideshow: 18 Ways NASA Uses Pi

Whether it's sending spacecraft to other planets, driving rovers on Mars, finding out what planets are made of or how deep alien oceans are, pi takes us far at NASA. Find out how pi helps us explore space.

Related Activities

educators.

The Sky and Dichotomous Key

Students learn about cloud types to be able to predict inclement weather. They will then identify areas in the school affected by severe weather and develop a solution to ease the impacts of rain, wind, heat or sun.

educators.

Precipitation Towers: Modeling Weather Data

This lesson uses stacking cubes as a way to graph precipitation data, comparing the precipitation averages and seasonal patterns for several locations.

educators.

Modeling the Water Budget

Students use a spreadsheet model to understand droughts and the movement of water in the water cycle.

students.

Make a Cloud Mobile - NASA SpacePlace

This mobile of feathery clouds will twist and turn in a gentle breeze. It even includes rain clouds with sparkling showers!

Multimedia

students.

Infographic: Planet Pi

This poster shows some of the ways NASA scientists and engineers use the mathematical constant pi (3.14) and includes common pi formulas.

Cloud Computing: A 'Pi in the Sky' Math Challenge

Overview

Materials

Background